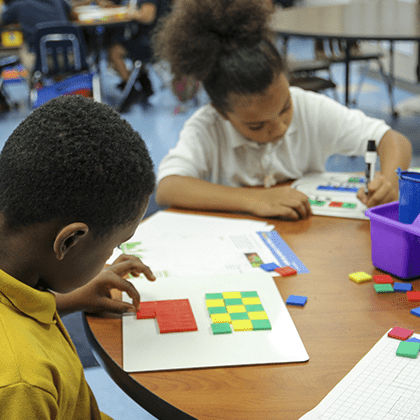
Trust Me: Using Multisensory Learning in Mathematics and Reading
2-MIN. READ
Find out how Liz's loss of taste and smell during the pandemic helped her teach students trust and conceptual understanding through multisensory learning.


Discover four strategies to maintain joy and perspective during high-stakes testing.

Discover creative ways to celebrate growth and demonstrate appreciation toward teachers.

Get tips, best practices, and success stories from other educators.
2-MIN. READ
Find out how Liz's loss of taste and smell during the pandemic helped her teach students trust and conceptual understanding through multisensory learning.
3-MIN. READ
Explore four key takeaways on how Curriculum Associates uses feedback to develop accessible products.
2-MIN. READ
Here are four tips on how to understand and teach mathematical instruction based on current strategies and standards.
2-MIN. READ
Find out how celebrating student growth, both big and small, fosters a love for learning in the classroom.
2-MIN. READ
Discover why using manipulatives in math is important.
2-MIN. READ
Implement these engaging games in the classroom to make building math fluency fun.
2-MIN. READ
Teachers are essential to student growth. Discover ways to celebrate and demonstrate their enormous contributions.
2-MIN. READ
Get tips for setting up math centers in your classroom to support student growth.
2-MIN. READ
Find out how building trust and creating a safe space helps you connect with middle schoolers and enhance student learning.
2-MIN. READ
Learn four strategies to maintain a positive perspective during high-stakes testing.
2-MIN. READ
Algebra-ready students will be prepared to succeed in high-level mathematics. Learn how to determine if your students are ready.
2-MIN. READ
Acceleration focused on content and timing allows the whole class to access grade-level content.